Eureka Math Grade 5 Module 3 Lesson 15 Answer Key (2024)

Engage NY Eureka Math 5th Grade Module 3 Lesson 15 Answer Key

Eureka Math Grade 5 Module 3 Lesson 15 Sprint Answer Key

A
Circle the Smaller Fraction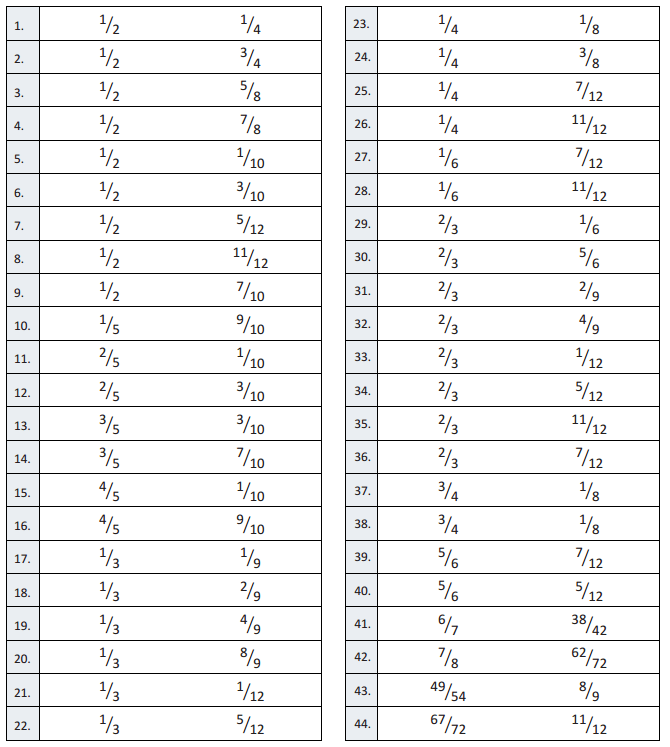

Question 1.
$\frac{1}{2}$ $\frac{1}{4}$
Answer: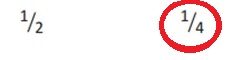

Question 2.
$\frac{1}{2}$ $\frac{3}{4}$
Answer:

Question 3.
$\frac{1}{2}$ $\frac{5}{8}$
Answer:

Question 4.
$\frac{1}{2}$ $\frac{7}{8}$
Answer:

Question 5.
$\frac{1}{2}$ $\frac{1}{10}$
Answer:

Question 6.
$\frac{1}{2}$ $\frac{3}{10}$
Answer:

Question 7.
$\frac{1}{2}$ $\frac{5}{12}$
Answer:

Question 8.
$\frac{1}{2}$ $\frac{11}{12}$
Answer:

Question 9.
$\frac{1}{2}$ $\frac{7}{10}$
Answer:

Question 10.
$\frac{1}{5}$ $\frac{9}{10}$
Answer:

Question 11.
$\frac{2}{5}$ $\frac{1}{10}$
Answer:

Question 12.
$\frac{2}{5}$ $\frac{3}{10}$
Answer:

Question 13.
$\frac{3}{5}$ $\frac{3}{10}$
Answer:

Question 14.
$\frac{3}{5}$ $\frac{7}{10}$
Answer:

Question 15.
$\frac{4}{5}$ $\frac{1}{10}$
Answer:

Question 16.
$\frac{4}{5}$ $\frac{9}{10}$
Answer:

Question 17.
$\frac{1}{3}$ $\frac{1}{9}$
Answer:

Question 18.
$\frac{1}{3}$ $\frac{2}{9}$
Answer:

Question 19.
$\frac{1}{3}$ $\frac{4}{9}$
Answer:

Question 20.
$\frac{1}{3}$ $\frac{8}{9}$
Answer:

Question 21.
$\frac{1}{3}$ $\frac{1}{12}$
Answer:

Question 22.
$\frac{1}{3}$ $\frac{5}{12}$
Answer:

Question 23.
$\frac{1}{4}$ $\frac{1}{8}$
Answer:

Eureka Math Grade 5 Module 3 Lesson 15 Problem Set Answer Key

Solve the word problems using the RDW strategy. Show all of your work.
Question 1.
In a race, the-second place finisher crossed the finish line 1$\frac{1}{3}$ minutes after the winner. The third-place finisher was 1$\frac{3}{4}$ minutes behind the second-place finisher. The third-place finisher took 34$\frac{2}{3}$ minutes. How long did the winner take?
Answer:
Fraction of time of Second place finisher crossed the line after = 1$\frac{1}{3}$ minutes = $\frac{4}{3}$
Fraction of time of Third place finisher is behind the second place = 1$\frac{3}{4}$ minutes = $\frac{7}{4}$
Fraction of time the third place finisher took = 34$\frac{2}{3}$ = $\frac{105}{3}$
Fraction of time the second place runner took = $\frac{105}{3}$ – $\frac{7}{4}$ = $\frac{420}{12}$ – $\frac{21}{12}$ = $\frac{399}{12}$ = $\frac{133}{4}$
Fraction of time the First place runner took = $\frac{133}{4}$ – $\frac{4}{3}$ = $\frac{399}{12}$ – $\frac{16}{12}$ = $\frac{383}{12}$ = 31 $\frac{11}{12}$ .
Therefore the First Runner took =31 $\frac{11}{12}$ .minutes.

Question 2.
John used 1$\frac{3}{4}$ kg of salt to melt the ice on his sidewalk. He then used another 3$\frac{4}{5}$ kg on the driveway. If he originally bought 10 kg of salt, how much does he have left?
Answer:
Fraction of Salt used by John =1$\frac{3}{4}$ kg = $\frac{7}{4}$ kg
Fraction of Salt used again =3$\frac{4}{5}$ kg = $\frac{24}{5}$ kg
Fraction of salt used = $\frac{7}{4}$ + $\frac{24}{5}$ = $\frac{35}{20}$ + $\frac{96}{20}$ = $\frac{131}{20}$ = 6 $\frac{11}{20}$ .
Total Salt = 10 kg.
Fraction of salt left = 10 – $\frac{131}{20}$ = $\frac{200}{20}$ – $\frac{131}{20}$ = $\frac{69}{20}$ = 3$\frac{9}{20}$ .
Therefore Fraction of salt left = 3$\frac{9}{20}$ .

Question 3.
Sinister Stan stole 3$\frac{3}{4}$ oz of slime from Messy Molly, but his evil plans require 6$\frac{3}{8}$ oz of slime. He stole another 2$\frac{3}{5}$ oz of slime from Rude Ralph. How much more slime does Sinister Stan need for his evil plan?
Answer:
Fraction of slime stolen from Messy Molly = 3$\frac{3}{4}$ = $\frac{15}{4}$ oz
Fraction of slime stolen from Messy Molly again = 2$\frac{3}{5}$ = $\frac{13}{5}$ oz
Total Fraction Stolen = $\frac{15}{4}$ + $\frac{13}{5}$ = $\frac{75}{20}$ + $\frac{52}{20}$ = $\frac{127}{20}$ = 6$\frac{7}{20}$ .
Fraction of more slime required = 6$\frac{3}{8}$ – $\frac{127}{20}$ = $\frac{51}{8}$ – $\frac{127}{20}$ = $\frac{255}{40}$ – $\frac{254}{40}$ = $\frac{1}{40}$ .
Therefore, Fraction of more slime required = $\frac{1}{40}$ oz.

Question 4.
Gavin had 20 minutes to do a three-problem quiz. He spent 9$\frac{3}{4}$ minutes on Problem 1 and 3$\frac{4}{5}$ minutes on Problem 2. How much time did he have left for Problem 3? Write the answer in minutes and seconds.
Answer:
Time given for 3 problems = 20 minutes
Fraction of time Spent on Problem 1 = 9$\frac{3}{4}$ minutes = $\frac{39}{4}$ .
Fraction of Time spent on Problem 2 = 3$\frac{4}{5}$ = $\frac{19}{5}$ .
Fraction of Time spent on Problem 3 = x
20 = $\frac{39}{4}$ + $\frac{19}{5}$ + x
x = 20 – $\frac{39}{4}$ – $\frac{19}{5}$
x = $\frac{400}{20}$ – $\frac{195}{20}$ – $\frac{76}{20}$
x = $\frac{129}{20}$ = 6$\frac{9}{20}$ .
Therefore, Fraction of Time spent on Problem 3 = 6$\frac{9}{20}$ .

Question 5.
Matt wants to shave 2$\frac{1}{2}$ minutes off his 5K race time. After a month of hard training, he managed to lower his overall time from 21$\frac{1}{5}$ minutes to 19$\frac{1}{4}$ minutes. By how many more minutes does Matt need to lower his race time?
Answer:
Fraction of Time lowered = 21$\frac{1}{5}$ minutes to 19$\frac{1}{4}$ minutes. = $\frac{106}{5}$ – $\frac{77}{4}$ = $\frac{424}{20}$ – $\frac{385}{20}$ = $\frac{39}{20}$ =1$\frac{19}{20}$ .
Fraction of Time shaved = 2$\frac{1}{2}$ =$\frac{5}{2}$ .
Fraction of More Time Matt need to lower his race time = $\frac{5}{2}$ – $\frac{39}{20}$ = $\frac{50}{20}$ – $\frac{39}{20}$ = $\frac{11}{20}$ = $\frac{33}{60}$ = 33 minutes .

Eureka Math Grade 5 Module 3 Lesson 15 Exit Ticket Answer Key

Solve the word problem using the RDW strategy. Show all of your work.
Cheryl bought a sandwich for 5$\frac{1}{2}$ dollars and a drink for $2.60. If she paid for her meal with a $10 bill, how much money did she have left? Write your answer as a fraction and in dollars and cents.
Answer:
Fraction of Cost of sandwich = 5$\frac{1}{2}$ = $\frac{11}{2}$ dollar = 5.5 dollar
Fraction of Cost of Drink = $2.60.
Total Cost = 5.5 +2.60 = 8.1 $.
Amount paid = 10$.
Money left = 10 – 8.1 = 1.9 $ .

Eureka Math Grade 5 Module 3 Lesson 15 Homework Answer Key

Solve the word problems using the RDW strategy. Show all of your work.
Question 1.
A baker buys a 5 lb bag of sugar. She uses 1$\frac{2}{3}$ lb to make some muffins and 2$\frac{3}{4}$ lb to make a cake. How much sugar does she have left?
Answer:
Total Quantity of Sugar = 5 lb
Fraction of Quantity of Suagr used for muffins = 1$\frac{2}{3}$ lb = $\frac{5}{3}$
Fraction of Quantity of Suagr used cake = 2$\frac{3}{4}$ lb = $\frac{11}{4}$
Fraction of Quantity of Sugar used = $\frac{5}{3}$ + $\frac{11}{4}$ = $\frac{20}{12}$ + $\frac{33}{12}$ = $\frac{53}{12}$
Fraction of Quantity of Sugar left = 5 – $\frac{53}{12}$ = $\frac{60}{12}$ – $\frac{53}{12}$ =$\frac{7}{12}$ .
Therefore, Fraction of Quantity of sugar left = $\frac{7}{12}$ .

Question 2.
A boxer needs to lose 3$\frac{1}{2}$ kg in a month to be able to compete as a flyweight. In three weeks, he lowers his weight from 55.5 kg to 53.8 kg. How many kilograms must the boxer lose in the final week to be able to compete as a flyweight?
Answer:
Fraction of weight need to lose in month = 3$\frac{1}{2}$ = $\frac{7}{2}$ = 3.5 kg
Weight lost in 3 weeks = 55.5 – 53.8 = 1.7 kg
Weight need to lose in final week = 3.5 – 1.7 = 1.8 kg.

Question 3.
A construction company builds a new rail line from Town A to Town B. They complete 1$\frac{1}{4}$ miles in their first week of work and 1$\frac{2}{3}$ miles in the second week. If they still have 25$\frac{3}{4}$ miles left to build, what is the distance from Town A to Town B?
Answer:
Fraction of work completed in first week = 1$\frac{1}{4}$ miles = $\frac{5}{4}$
Fraction of work completed in second week = 1$\frac{2}{3}$ miles = $\frac{5}{3}$
Fraction of work left to built = 25$\frac{3}{4}$ miles = $\frac{103}{4}$
Fraction of Distance from Town A to Town B = $\frac{103}{4}$ + $\frac{5}{4}$ + $\frac{5}{3}$ = $\frac{108}{4}$ + $\frac{5}{3}$ = $\frac{324}{12}$ + $\frac{20}{12}$ = $\frac{344}{12}$= 28$\frac{2}{3}$ .
Therefore, Fraction of Distance from Town A to Town B = 28$\frac{2}{3}$ miles.

Question 4.
A catering company needs 8.75 lb of shrimp for a small party. They buy 3$\frac{2}{3}$ lb of jumbo shrimp, 2$\frac{5}{8}$ lb of medium-sized shrimp, and some mini-shrimp. How many pounds of mini-shrimp do they buy?
Answer:
Quantity of shrimp needed = 8.75 lb =8$\frac{3}{4}$ = $\frac{27}{4}$
Quantity of jumbo shrimp = 3$\frac{2}{3}$ lb = $\frac{11}{3}$
Quantity of medium – sized shrimp = 2$\frac{5}{8}$ lb = $\frac{21}{8}$
Quantity of mini shrimp = x
$\frac{35}{4}$= $\frac{11}{3}$ + $\frac{21}{8}$ + x
x = $\frac{210}{24}$ – $\frac{88}{24}$ – $\frac{63}{24}$
x = $\frac{59}{24}$ = 2 $\frac{11}{24}$
Therefore, Quantity of mini shrimp = x = 2 $\frac{11}{24}$ lb .

Question 5.
Mark breaks up a 9-hour drive into 3 segments. He drives 2$\frac{1}{2}$ hours before stopping for lunch. After driving some more, he stops for gas. If the second segment of his drive was 1$\frac{2}{3}$ hours longer than the first segment, how long did he drive after stopping for gas?
Answer:
Total time of the drive = 9 hours .
Fraction of Time drived for first segment = 2$\frac{1}{2}$ hours = $\frac{5}{2}$
Fraction of Time of second segment = 1$\frac{2}{3}$ hours longer than the first segment = $\frac{5}{3}$ + $\frac{5}{2}$ = $\frac{10}{6}$ + $\frac{15}{6}$ = $\frac{25}{6}$ =4$\frac{1}{6}$
Fraction of Time of first and second segment= $\frac{5}{2}$ + $\frac{25}{6}$ = $\frac{15}{6}$ + $\frac{25}{6}$ = $\frac{40}{6}$ = 6$\frac{4}{6}$
Fraction of Time he drive after stopping gas = 9 – $\frac{40}{6}$ = $\frac{54}{6}$ – $\frac{40}{6}$ = $\frac{14}{6}$ = 2$\frac{2}{6}$ .
Therefore, Fraction of Time he drive after stopping gas = third segment = 2$\frac{2}{6}$ hours .

Eureka Math Grade 5 Module 3 Lesson 15 Answer Key (2024)

FAQs

What grade does Eureka math go up to? ›

Eureka Math^® is a holistic Prekindergarten through Grade 12 curriculum that carefully sequences mathematical progressions in expertly crafted modules, making math a joy to teach and learn. We provide in-depth professional development, learning materials, and a community of support.

Read The Full Story ›

What are the four core components of a Eureka Math TEKS lesson? ›

Each lesson in A Story of Units is comprised of four critical components: fluency practice, concept development (including the problem set), application problem, and student debrief (including the Exit Ticket).

Show Me More ›

Is Eureka Math a curriculum? ›

An Elementary, Middle, And High School Math Curriculum. Eureka Math^® is a math program designed to advance equity in the math classroom by helping students build enduring math knowledge.

What is the hardest math in 5th grade? ›

Some of the hardest math problems for fifth graders involve multiplying: multiplying using square models, multiplying fractions and whole numbers using expanded form, and multiplying fractions using number lines.

What is the hardest math grade? ›

Generally speaking, the most rigorous math courses in high school include Advanced Placement (AP) Calculus AB and BC, AP Statistics, and for some, Multivariable Calculus (which might be offered at your school or at a local college).

How long does an Eureka math lesson take? ›

Eureka Math is 1 hour for all grade levels (except in Kindergarten lessons are 50 minutes). We have always designed our elementary day with 1 hour dedicated to mathematics instruction. With Eureka Math, this is a dynamic hour of instruction with many different interactive components including student collaboration.

Get More Info Here ›

Is Eureka math aligned with TEKS? ›

The K-5 Math COVID Emergency Release Pilot Edition V2 is currently called Eureka Math TEKS Edition and was built based on Texas standards. Students develop solid conceptual understanding, practice procedural skills and fluency, and apply what they learn in real-world scenarios.

Read On ›

Is Eureka math the same as common core? ›

Eureka Math is a Common Core math. Eureka Math's framework is entirely built on the Common Core Learning Standards and Progressions for the Common Core State Standards in Mathematics.

Discover More Details ›

Is Eureka Math scripted? ›

Eureka Math is not intended to be followed as a script, instead as a guide to offer support to teachers in the classroom. For example, the “vignettes” of teacher-student interactions included in Eureka Math are exemplars of instructional situations provided by the teachers who have crafted our curricula.

Who is the owner of Eureka Math? ›

LYNNE MUNSON

At the urging of educators seeking knowledge-building resources, Lynne and her team moved into curriculum development with Common Core curriculum maps in English language arts and then with EngageNY, which later became Eureka Math^®.

Explore More ›

What is the highest level of math in 9th grade? ›

9th grade math usually focuses on Algebra I, but can include other advanced mathematics such as Geometry, Algebra II, Pre-Calculus or Trigonometry.

Tell Me More ›

What grade level does prodigy math go up to? ›

With 1,500+ curriculum-aligned math skills for 1st to 8th grade, Prodigy Math is so much more than a game. Prodigy Math is an engaging game-based learning platform that's dedicated to improving students' confidence and achievements in math.

View Details ›

What is advanced math in 8th grade? ›

Almost every school district in the state offers an accelerated math option for selected students. These students take Algebra I in 8th grade. These students complete Algebra II, Geometry and Precalculus one year earlier than their peers. This allows them to take AP Calculus A/B in their senior year.